Hard sudoku puzzles @ thesudoku.app
In addition to the techniques described in easy and medium sudoku tutorials, to solve hard sudoku puzzles @ thesudoku.app, you will need to use additional solving techniques, that will help you reduce the number of pencil marks and solve puzzle step by step:
- Intersection removal strategy («pointing pairs» and «box/line reduction»)
- «Naked quad»
- «Hidden pair» and «hidden triple»
Pointing pairs
If a candidate is present only in two or three cells of a 3x3 unit, and these cells belong to the same row or column, then this candidate cannot be a solution in other cells of this row or column respectively.
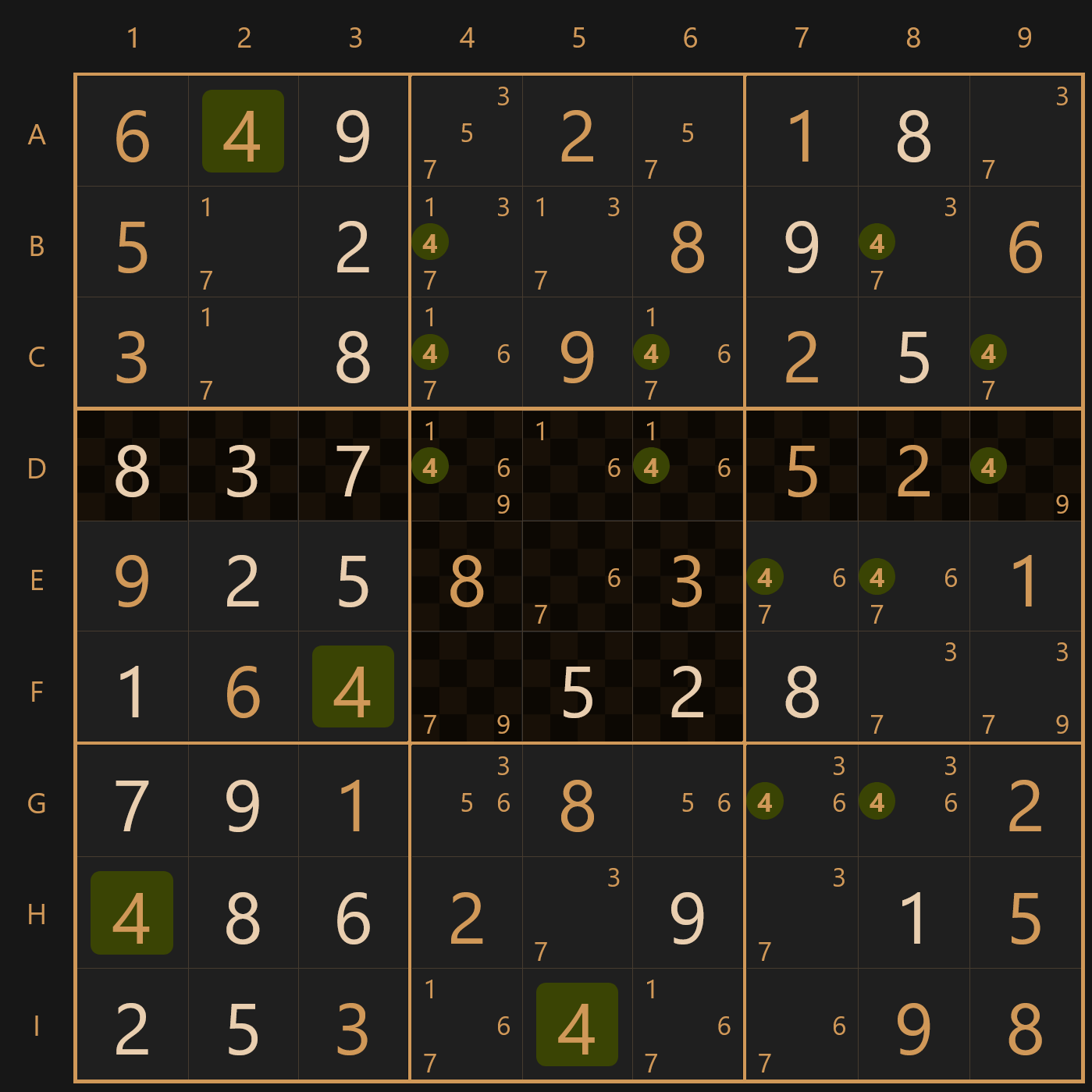
Pointing pair in a row
Take a look at the highlighted 3x3 unit. Candidate 4 can only be found in cells D4 and D6 within one row D. This means that you can eliminate candidate 4 from other cells of this row. After eliminating candidate 4 from the cell D9, the only candidate for this cell will be 9.
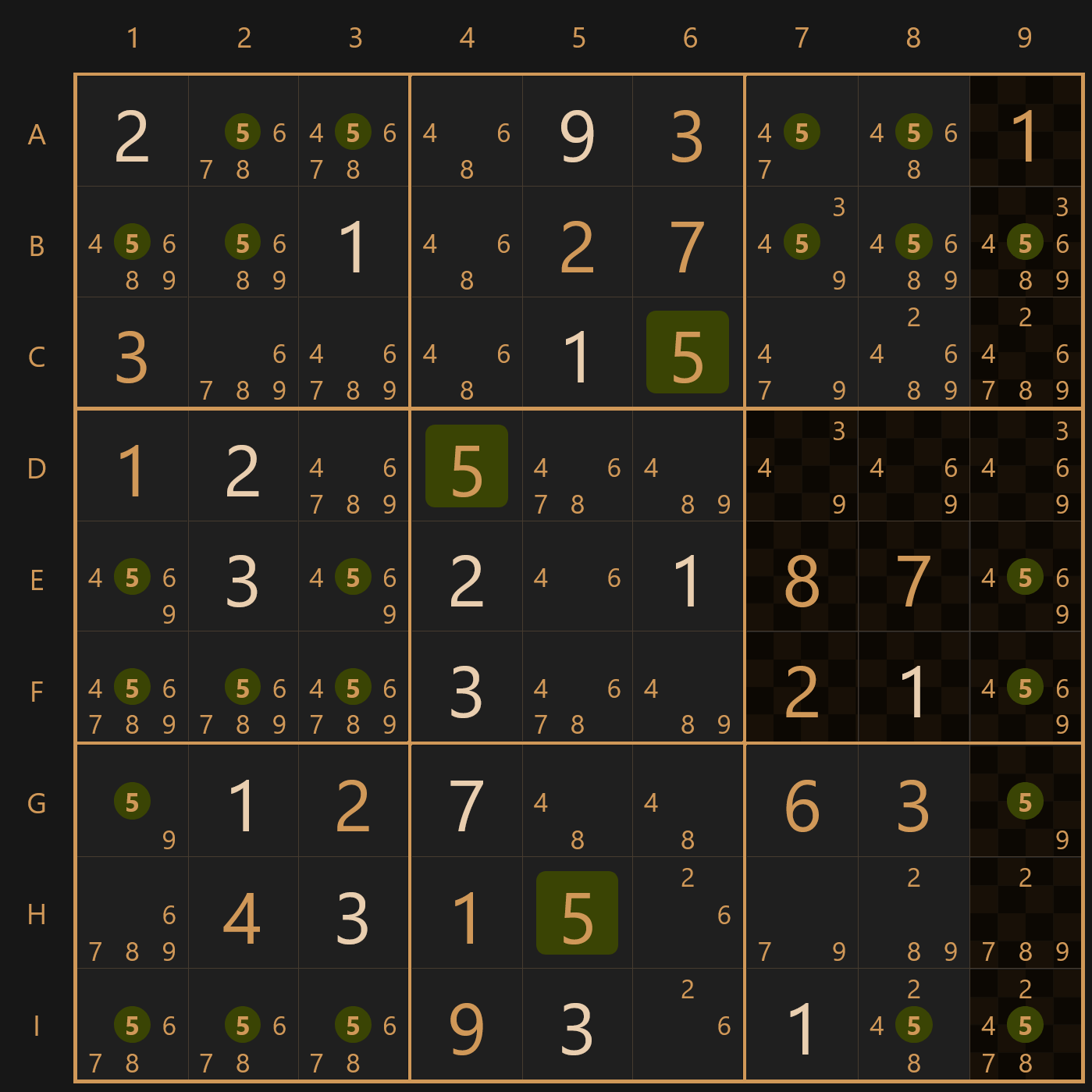
Pointing pair in a column
Take a look at the highlighted 3x3 unit. Candidate 5 can only be found in cells E9 and F9 within one column 9. This means that you can eliminate candidate 5 from other cells of this column. After eliminating candidate 5 from the cell G9, the only candidate for this cell will be 9.
Box/line reduction
This solving technique is very similar to the pointing pairs. If a candidate is present only in two or three cells of a row or column, and these cells belong to the same 3x3 unit, then this candidate cannot be a solution in other cells of this 3x3 unit.
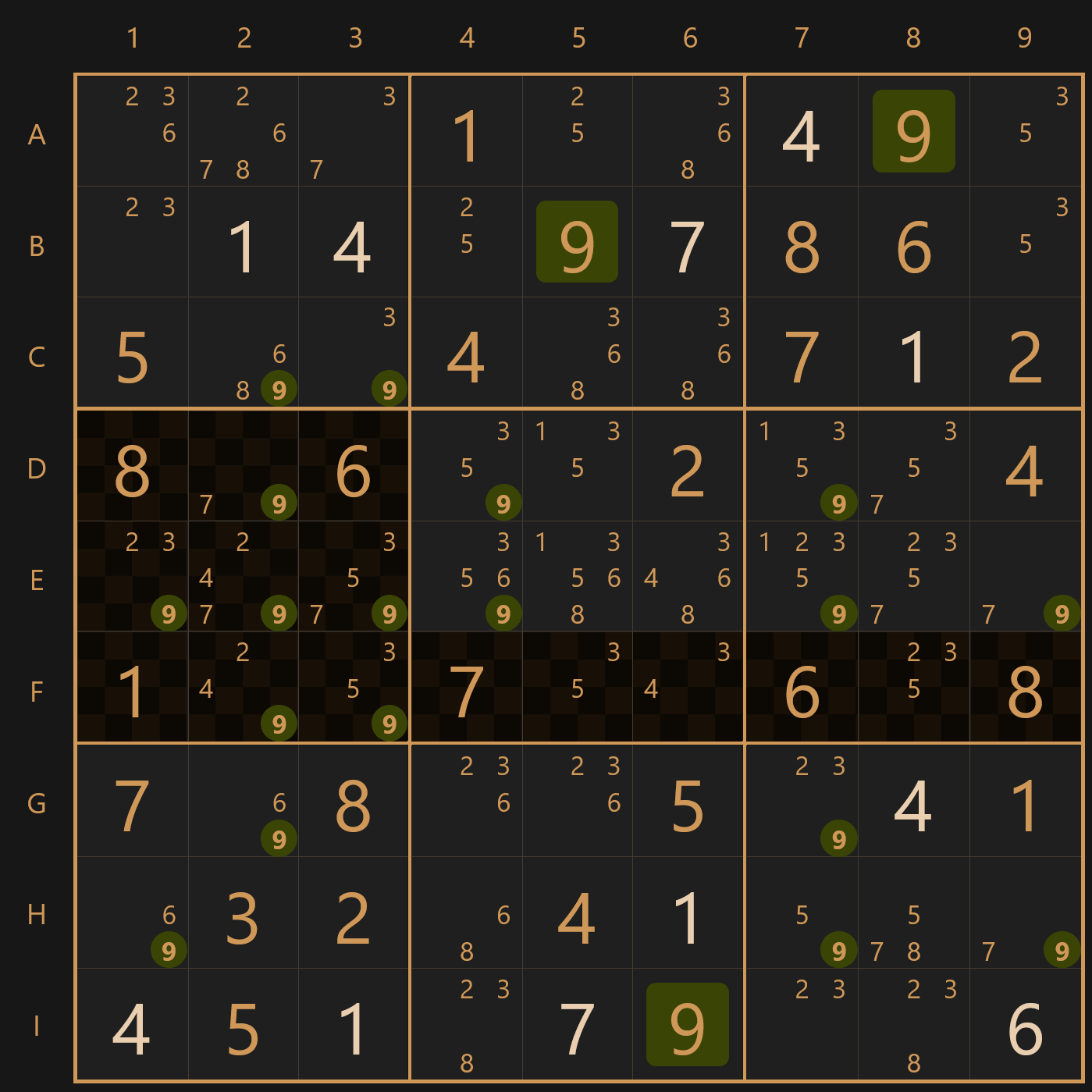
Box/line reduction in a row
Take a look at the highlighted row F. Candidate 9 can only be found in cells F1 and F3 within one highlighted 3x3 unit. This means that you can eliminate candidate 9 from other cells of this 3x3 unit. After eliminating candidate 9 from the cell D2, the only candidate for this cell will be 7.
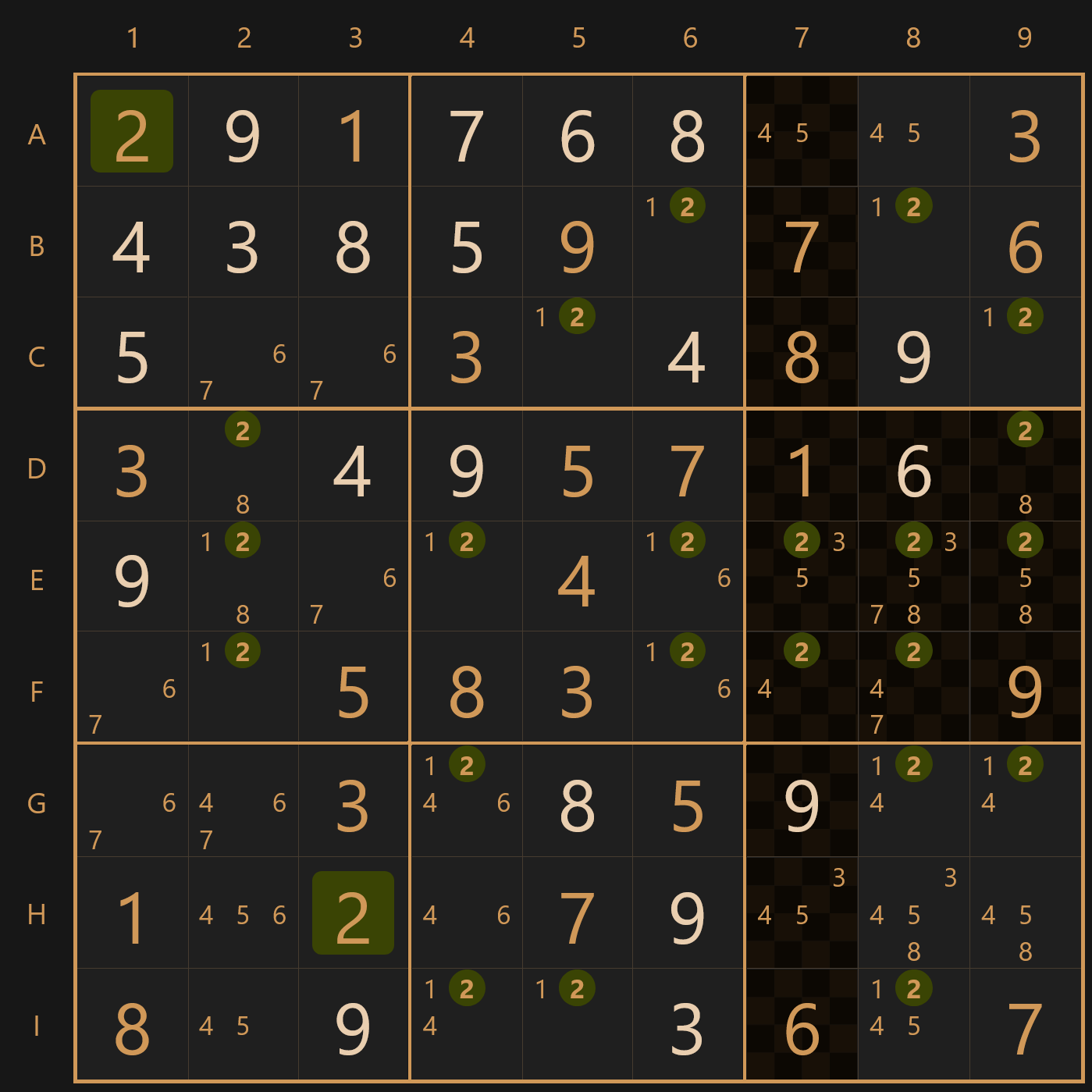
Box/line reduction in a column
Take a look at the highlighted column 7. Candidate 2 can only be found in cells E7 and F7 within one highlighted 3x3 unit. This means that you can eliminate candidate 2 from other cells of this 3x3 unit. After eliminating candidate 2 from the cell D9, the only candidate for this cell will be 8.
Naked quads
This strategy is a logical extension of the nakes pairs and nakes triples solving techniques, although it is less common.
A «naked quad» is four cells from the same row, column or 3x3 unit, which have same four candidates left in different combinations. Since none of these candidates can be the solution in any other cell in this row, column or 3x3 unit respectively, you can remove pencil marks from the other cells.
Take a look at the highlighted column 9. Cells D9, E9, F9 and G9 share same four candidates 4, 5, 6 and 9 - it is a naked quad. This means you can remove pencil marks 4, 5 and 9 from the cell A9, the only possible candidate for this cell will be 7.
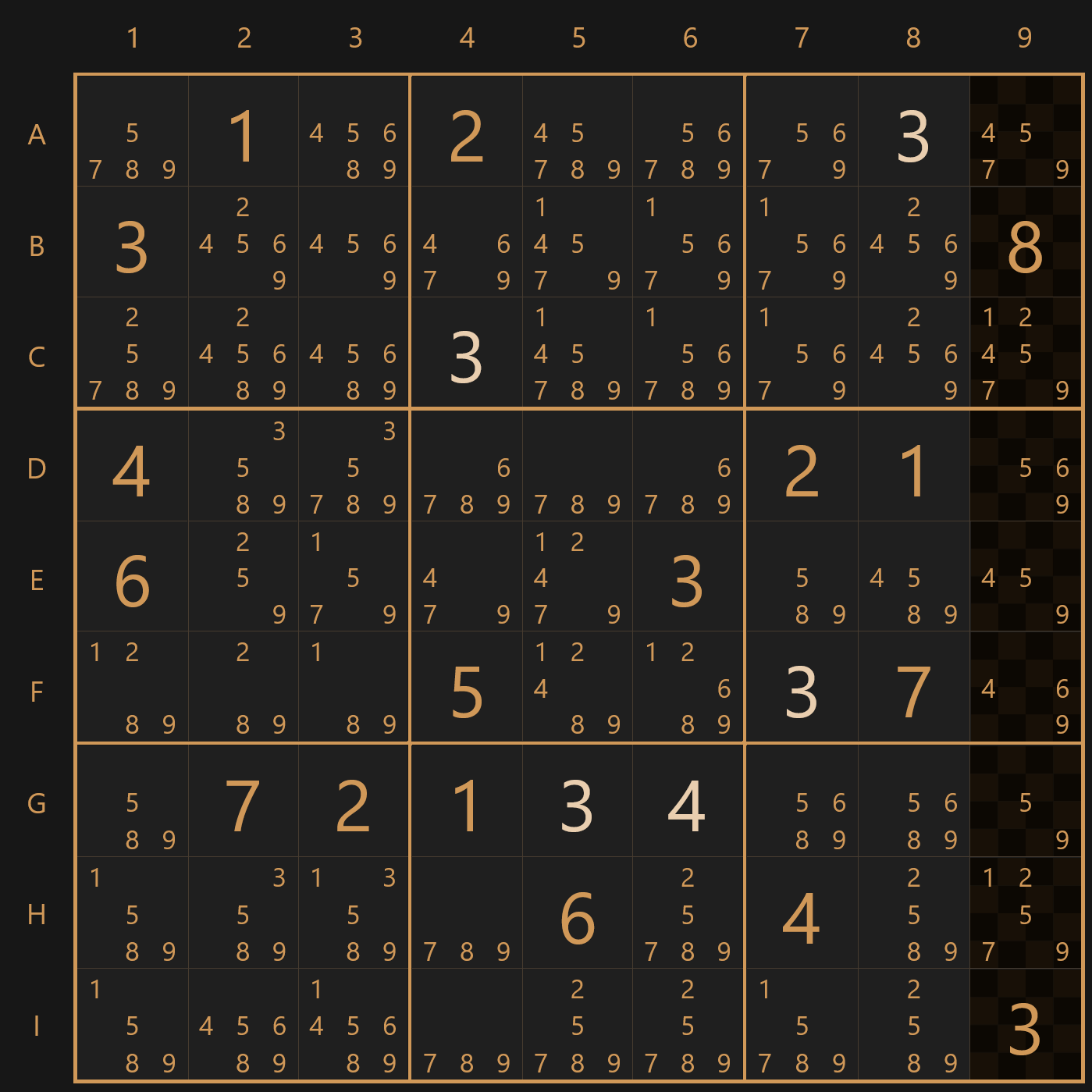
Hidden pairs
«Hidden pairs» solving technique is similar to naked pairs, except it's much harder to spot. If you feel stuck, you can use thesudoku.app smart hint feature.
If two candidates can be found only in two cells of the same row, column or 3x3 unit, then one of these candidates is the solution for one of these cells and the other candidate is the solution for the other cell. So you can eliminate the rest of the candidates from these cells
Take a close look at the highlighted 3x3 unit. Candidates 2 and 7 are located only in cells B8 and C7. So you can remove all other candidates from these cells.
Look at the hightlighted row B. After you eliminate candidate 1 from the cell B8, only one cell is left to place candidate 1 within this row, cell B2. It's a hidden single.
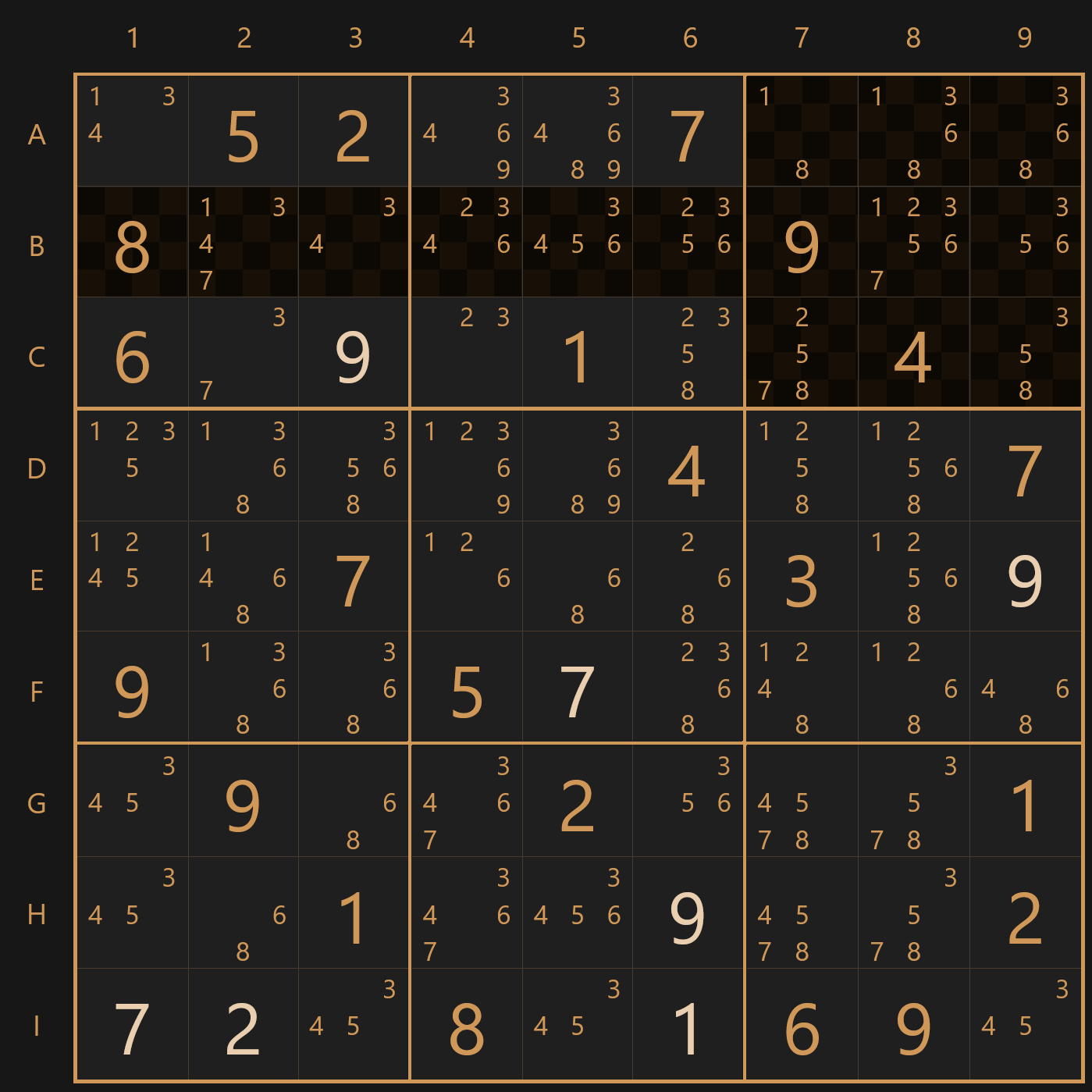
Hidden triples
«Hidden triples» is the same as hidden pairs, except there are three cells and three candidates involved.
This is an extremely rare solving technique. In fact, there is only one sudoku puzzle @ thesudoku.app that cannot be solved without using this technique.
Take a close look at the highlighted 3x3 unit. Candidates 1, 4 and 6 are located only in cells H4, H5 and I4. So you can remove all other candidates from these cells.
